Bài tập tuần 2 – Toán lớp 9

BÀI TẬP TUẦN 2

– Liên hệ giữa phép nhân (phép chia ) và phép khai phương

– 1 số hệ thức cạnh và đường cao trong tam giác vuông
Bài 1: Tính
a) $ \displaystyle \sqrt{90.360}$

b) $ \displaystyle \sqrt{52}.\sqrt{13}$

c) $ \displaystyle \sqrt{\frac{{{65}^{2}}-{{52}^{2}}}{196}}$

d) $ \displaystyle \frac{\sqrt{13}}{\sqrt{208}}$

Bài 2: Thực hiện phép tính
a) $ \displaystyle \sqrt{1,6}.\sqrt{250}+\sqrt{19,6}:\sqrt{4,9}$

b)$ \displaystyle \left( \sqrt{\frac{2}{3}}+\sqrt{\frac{50}{3}}-\sqrt{24} \right).\sqrt{6}$

c) $ \displaystyle \left( \sqrt{\frac{1}{3}}-\sqrt{\frac{4}{3}}+\sqrt{3} \right):\sqrt{3}$

d) $ \displaystyle \sqrt{3+\sqrt{5}}.\sqrt{2}$

Bài 3: Rút gọn biểu thức:
a) $ \displaystyle \frac{\sqrt{10}-\sqrt{15}}{\sqrt{8}-\sqrt{12}}$

b) $ \displaystyle \frac{5+\sqrt{5}}{\sqrt{10}+\sqrt{2}}$

c) $ \displaystyle \frac{x\sqrt{y}+y\sqrt{x}}{x+2\sqrt{xy}+y}$( với x ≥ 0, y ≥ 0, xy ≠ 0

d) $ \displaystyle \frac{x\sqrt{x}+y\sqrt{y}}{x-\sqrt{xy}+y}$

Bài 4: Rút gọn biểu thức sau:
a) A = $ \displaystyle \sqrt{8+2\sqrt{15}}-\sqrt{8-2\sqrt{15}}$

b) B = $ \displaystyle \sqrt{3-\sqrt{5}}-\sqrt{3+\sqrt{5}}$( 2 cách)

c*) C =$ \displaystyle \sqrt{2+\sqrt{3}}.\sqrt{2+\sqrt{2+\sqrt{3}}}.\sqrt{2+\sqrt{2+\sqrt{2+\sqrt{3}}}}.\sqrt{2-\sqrt{2+\sqrt{2+\sqrt{3}}}}$

d) $ \displaystyle \frac{\sqrt{15}-\sqrt{5}}{\sqrt{3}-1}+\frac{5-2\sqrt{5}}{2\sqrt{5}-4}$

e) $ \displaystyle \frac{3+2\sqrt{3}}{\sqrt{3}}+\frac{2+\sqrt{2}}{\sqrt{2}+1}-(\sqrt{2}+\sqrt{3})$

Bài 5: Tìm x, biết:

a) $ \displaystyle \sqrt{x-5}+\sqrt{4x-20}-\frac{1}{5}\sqrt{9x-45}=3$

b) $ \displaystyle 2\sqrt{9x-27}-\frac{1}{5}\sqrt{25x-75}-\frac{1}{7}\sqrt{49x-147}=20$

c) $ \displaystyle \sqrt{{{x}^{2}}-2x+9}=2x-3$

d)$ \displaystyle \sqrt{6-2{{x}^{2}}}+1=x$

e) $ \displaystyle \sqrt{x-3}-2\sqrt{{{x}^{2}}-9}=0$

f) $ \displaystyle \frac{\sqrt{x-3}}{\sqrt{2x+1}}=2$

Bài 6*: Tìm giá trị lớn nhất của biểu thức

a) A = $ \displaystyle \frac{\sqrt{{{x}^{2}}+x+3}}{\sqrt{{{x}^{2}}+x+1}}$

b) B = $ \displaystyle \sqrt{1-x}.\sqrt{x+3}$

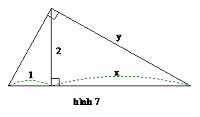
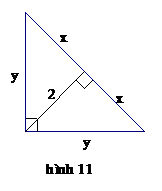
Bài 7: Tìm x, y có trên hình vẽ sau :

Bài tập tuần 2 - Toán lớp 9

Bài 9*: Cho tam giác ABC cân tại A, đường cao AH và BK. Chứng minh:

$ \displaystyle \frac{1}{B{{K}^{2}}}=\frac{1}{B{{C}^{2}}}+\frac{1}{4A{{H}^{2}}}$

Bài cùng series: